ICTS-NETWORKS workshop: A Confluence of Ideas
In the vibrant city of Bangalore, India, the International Centre for Theoretical Sciences (ICTS) played host to a discussion meeting titled "Challenges in Networks" from January 29 to February 2, 2024.
Does no small structure mean larger homogeneous ones?
A conjecture of Erdős and Hajnal from 1989 says that forbidding any specific substructure results in existence of a very large homogeneous one! In this article you will have a look into one of the most fascinating problems in modern graph theory.
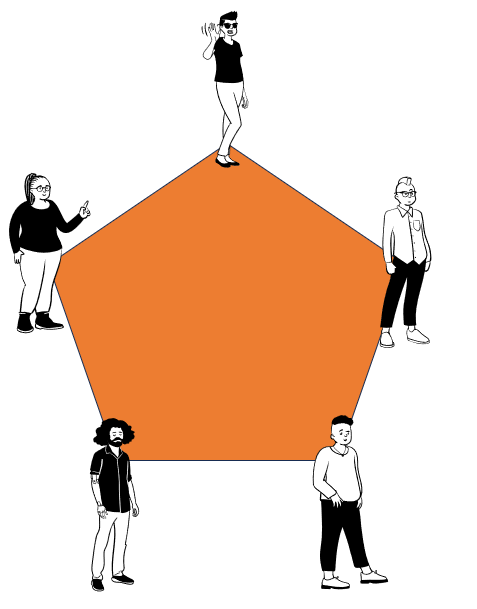
Picking up 13 different cards from 13 piles (Part 2)
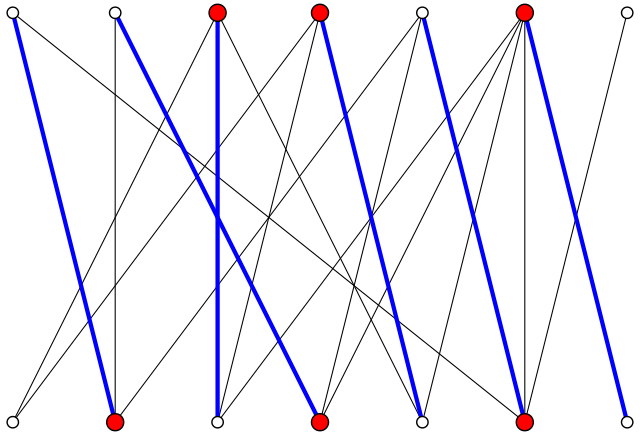
In Part 1 Jackie explained to her fried Sam how the problem of picking a card from each of the 13 piles so that there is exactly one card with each rank translates to a problem on bipartite graphs. The mathematical problem asks you to find a perfect matching in a regular bipartite graph.
Picking up 13 different cards from 13 piles (Part 1)
Did you know that if you divide a pack of cards into 13 piles of 4 cards, then you can always pick one card from each of the 13 piles so that there is exactly one card with each rank? There is some beautiful math behind this puzzle.
The 100 prisoners escape puzzle
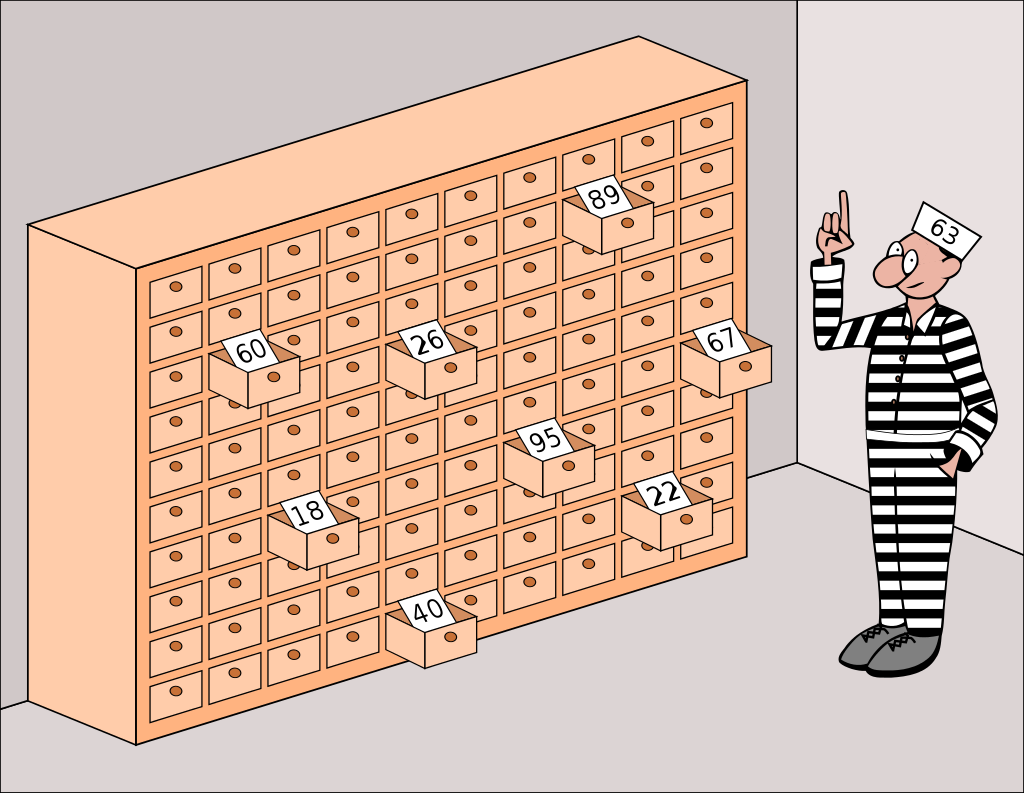
In this article, we will discuss a mathematical riddle that "seems impossible even if you know the answer". It is better known as the 100 prisoners problem.
Structure is everywhere. So is chaos.
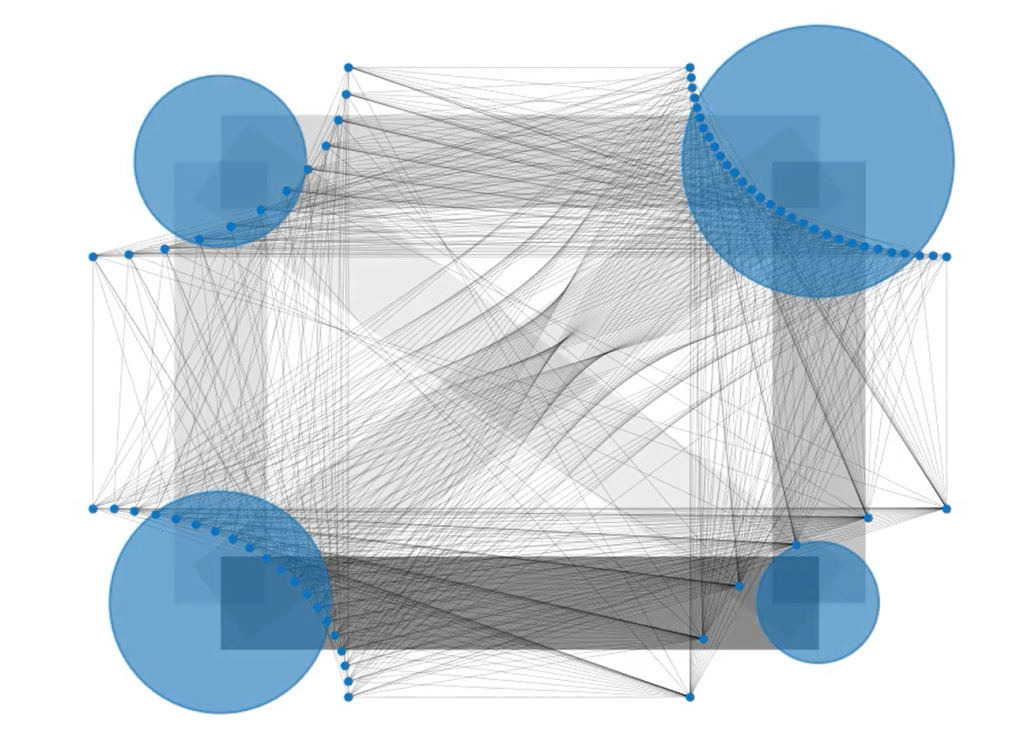
Pick 45 numbers between 1 and 100. Try to avoid creating pairs whose difference is the square of some number and you will fail. Always.
Is a rapidly mutating virus unstoppable?
How can one hope to understand the precise structure of a virus if it is able to become unrecognizable within weeks? The mathematics behind this questions didn't let go of my mind for extensive periods of time during my PhD studies in Belgium.
Is it easier to find half a needle than a full needle in a random network?
Common sense tells us that objects of comparable size should be equally hard to find. Yet, when searching inside a random network, surprises are awaiting . . .
New breakthrough about Ramsey numbers?
In a seminar talk in Cambridge this week, Julian Sahasrabudhe announced that he, together with his colleagues Marcelo Campos, Simon Griffiths and Rob Morris, had obtained an exponential improvement to the upper bound for Ramsey's theorem.
AI thinks my dog is a Pig! Want to know why?
Applications of machine learning models are everywhere, with many online platforms and major science fields using tools relying on machine learning. Take, for example, image recognition and computer vision. But did you know that the results of supposedly perfect and accurate machine learning models can be deceived by slight perturbations in the data?