After having a near-fatal accident falling off his horse, Prussian footman Hans Berger (1873—1941) was surprised to learn that his sister had sent—being kilometers away—him a telegram to let him know that she sensed he was in grave danger.
Berger was convinced that he and his sister had communicated telepathically through “psychic energy”. Since he was a trained physician, this event led him to search for the scientific basis of telepathy: Berger suspected that the electrical activity of the brain could cause large enough disruptions in the magnetic field so as to influence the brains of others.
Although this theory was quickly dismissed, Berger found that the electrical activity in the brains of subjects with their eyes closed showed characteristic periodic oscillatory behavior. Even more fascinating, these oscillations would cease as soon the subjects opened their eyes. These discoveries were poised to stay: The oscillations, or brain waves, Berger discovered operated at frequencies around 10Hz and are now referred to as alpha waves. Moreover, the technique of measuring electrical activity by placing electrodes on the skull is now known as “electroencephalography” and is a common approach to noninvasively measure brain dynamics. To date, electroencephalography is widely used to detect and treat diseases such as epilepsy and sleep disorders.
Remarkably, our current understanding of alpha waves is very much consistent with Berger's discoveries in the 1930s. The opening of eyes, mental activity, visual imagination, visual stimuli, and acoustic stimuli are all events that result in decrease in alpha oscillations. Consequently, researchers strongly believe that alpha oscillations are tightly involved in idle (inactive yet awake) mental behaviour.
Since Berger's work, brain waves other than alpha-waves have been characterized and are typically labelled by their frequency: delta (0.5—4Hz), theta (4—8Hz), alpha (8—12Hz), beta (12—30Hz), and gamma (30—120Hz). While alpha-waves are associated with idle brain dynamics, brain oscillations in other frequency bands have been connected to various cognitive brain functions such as memory formation, concentration, and intelligence.
Thus, it is generally thought that brain oscillations play an important role in the functioning of the brain. Therefore, the neuroscientific community have been researching brain waves intensely over the past decades. While brain waves can be measured in patients, mathematical models allow to get insights how oscillations emerge in the brain.
The computational modeling of neurons and neuronal networks has a long history, dating back to the Nobel Prize awarded work by Huxley and Hodgkin in 1963. Huxley and Hodgkin studied individual giant squid neurons and modeled their activity with high precision. By contrast, the brain waves discovered by Berger do not involve single neurons but reflect the synchronized activity of tens of thousands of neurons.
To understand the joint activity of large populations of interconnected neurons, Wilson and Cowan made a groundbreaking contribution in a 1972 paper, by creating a model that describes the average behaviour of many neurons simultaneously. The model equations that describe a population of many neurons—typically called a neural mass—can reproduce the rhythmic activity first observed by Berger. As such, these networks can be used to model the brain waves at specific brain regions, or even the whole human brain.
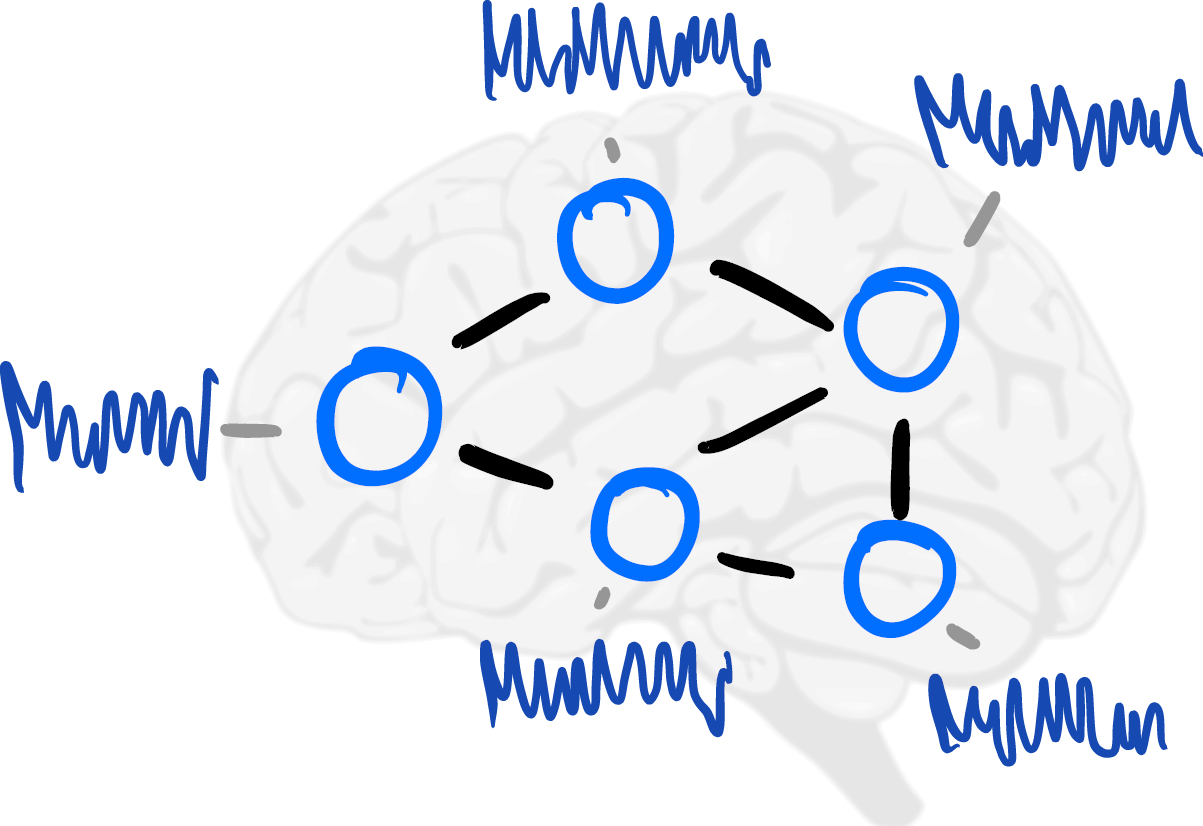
Such simple models are building blocks to create dynamical models (mathematical models which can be used to study the evolution of a system in time) that capture the rhythmic activity of the entire brain. Researchers can measure the connectivity between different brain regions to reveal how different areas of the brain are connected as a network. If each region is then represented by a building block consisting of a neural mass, we obtain a dynamical model for the brain as a network of coupled neural masses.
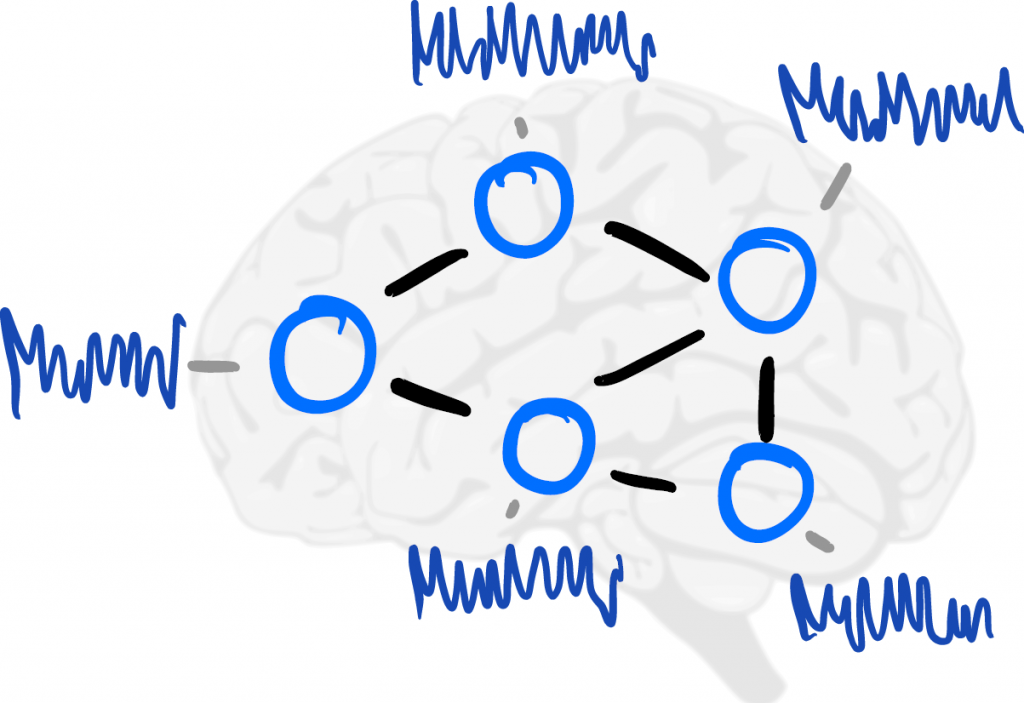
A graphical representation of a network of neural masses.
Such network models can not only reproduce brain activity but are also very helpful to predict the effect of brain disruptions (whether through the growth of a tumor or a man-made intervention such as surgery) on brain dynamics, such as brain oscillations. With more detailed models being developed every day, the hope is to eventually be able to predict treatment: Can we reliably address the effect of brain surgery and medication in simulations without actually performing the surgery itself?
Neurological diseases such as Parkinson's disease, schizophrenia, depression, epilepsy, and Alzheimer's disease affect cognitive function. This is reflected in the disturbance of healthy brain wave activity that one can measure. But how does disease-related damage to neurons lead to disruption of brain waves, which arise from the coordinated activity of hundreds of thousands of such cells?
For example, in Alzheimer's disease, two types of toxic proteins have been identified that spread in a distinct fashion across the brain. These two proteins affect neurons in different ways, but are both debilitating to normal neuronal activity. Meanwhile, clinicians also observe changes to the brain waves. Specifically, they observe that the alpha frequency, first discovered by Berger, decrease in strength and slows down. However, what causes these changes in the alpha brain waves is not clear yet.
Using neural mass models of the brain, researchers can now try to understand how brain rhythms change during diseases like Alzheimer's. For example, in Alzheimer's disease, different hypotheses for how the disease develops can be tested by simulating Alzheimer's on a neural mass brain model. Doing so, researchers can find more plausible hypotheses for why the alpha frequency slows down in Alzheimer's patients.
Another important issue is that of early detection of brain diseases. By simulating brain disease on a neural mass network, researchers can find potential early signs of disease. Additionally, the effects of medicine can be implemented into computational brain simulations and researchers can analyze what kind of medicine will be the most effective in battling different kinds of brain disease.
Current modelling efforts of brain waves and how they change in disease often focus on dynamics within a single frequency band. However, to fully understand the nature of brain waves, researchers will have to grapple with all the brain wave frequencies, not just the alpha frequency. In particular, the network mechanisms of how brain waves at different frequencies interact and affect brain function is yet to be fully understood.
Further research into such cross-frequency interaction will open avenues for a deeper understanding of brain diseases, as they often feature complicated changes across frequencies. Including interactions between frequencies is just one step of many towards a full reconstruction of brain dynamics. New neural mass models able to explain complicated dynamics occurring during sleep, epilepsy, and memory formation are also being researched at the moment.
Hopefully, researchers will soon piece together this puzzle and be able to faithfully simulate the human brain, helping us to fix it once it becomes broken.
Synchronization is a phenomenon which has received a lot of attention in the last few decades. It is the mechanism by which a group of individuals that go through some periodic motion begin moving through the same parts of their cycles at the same time.
The part of the brain that controls the sleeping cycle is made up of two groups of neurons. In order for you to be able to sleep at night, the activity of these neurons needs to be synchronized with the cycle of the sun (e.g. active during the day and inactive at night). When you abruptly change the cycle of the sun (by flying to New York) these two groups need to re-synchronize to the new sun-cycle.
The body-clock, which is a cluster of neurons in the brain, has the same structure in all mammals, which is remarkable. It consists of two groups: two-communities of neurons that are strongly linked within each community and less strongly linked between the communities.
As many phenomena in the classical world have quantum counter part, it raises the question if this is also true for synchronization. As synchronization is a collective phenomenon, it may help gaining a better understanding of how collective phenomena in the classical and quantum world are connected.
