The Christiaan Huygens Science Prize honors a young researcher who has made an innovative contribution to astronomy, mathematics or physics in their dissertation. The prize has been awarded annually since 1998. In 2024, the field of mathematics was central. The prize was awarded on the 16th of October to mathematician Jan Willem van Ittersum, who completed his PhD three years ago at Utrecht University.
Jan-Willem van Ittersum received the Christiaan Huygens Science Prize. Rosa Schwartz and Lucas Slot received an honorary distinction. The photo is taken from the website of the organization.
On the website of the event we read:
"Researcher Jan Willem van Ittersum has received the Christiaan Huygens Science Prize for his dissertation "Partitions and quasi-modular forms; variations on the Bloch-Okounov theorem". He received the prize - consisting of an amount of 10 thousand euros and a bronze statue by Christiaan Huygens - on October 16, 2024 from Minister Bruins of Education, Culture and Science. This happened during a festive meeting in Voorburg.
Counting the number of different solutions to a problem, or the number of possible configurations in a model, is an important challenge in mathematics and physics. The Bloch–Okounkov theorem shows that these types of counting problems sometimes exhibit underlying extra symmetry, which is called modular symmetry. That is an important insight which can help us better understand the original problem.
Van Ittersum's dissertation provides a completely new view of the Bloch-Okounkov theorem, namely through the lens of the theoretical theory of modular forms. Modular forms are certain types of functions, which have been studied extensively by mathematics and are very well understood. Van Ittersum's study leads to important extensions of the Bloch-Okounkov theorem, and to new applications at the intersection of algebraic geometry, number theory and representation theory, with important connections to statistical physics."
The jury, chaired by Eric Opdam, professor of mathematics at the University of Amsterdam, was deeply impressed by the quality and innovative contribution of the dissertation to the field of mathematics.
Van Ittersum presents spectacular new results on profound topics in mathematics
according to the jury report.
He not only shows virtuosity in connecting different concepts and theories at a high level of abstraction, but at the same time ensures a clear and accessible presentation of these ideas.
Van Ittersum is now regarded worldwide as an expert in the methodology described in the thesis.
Understanding partitions
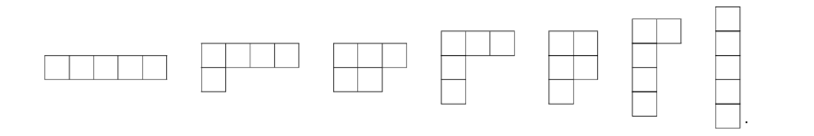
Central to van Ittersum's research is the concept of a partition of a number. A partition of an integer is a way to express the number as a sum of positive integers, where the order of the terms does not matter. For example, the partitions of 5 are given by 5, 4+1, 3+2, 3+1+1, 2+2+1, 2+1+1+1, and 1+1+1+1+1, which can be represented graphically by their Young diagrams as
Partitions of numbers have intrigued mathematicians since the 18th century. For instance, how can you find all partitions of a number? Is there a systematic way to do this? Such questions quickly proved to be very challenging, even for today’s most powerful computers. To get an idea: the number 100 has 190,569,292 partitions. If you try to find them all then you are probably doomed to make a mistake, or get exhausted and never want to do math again!
Mathematicians therefore had to be creative in order to better understand partitions. The famous mathematician Leonard Euler (1707–1783) was the first to use functions to study partitions. He was the first to develop a method to determine the number of partitions of a number by using derivatives of a well-chosen function. In 1918, mathematicians G.H. Hardy and S. Ramanujan introduced an approach to estimate the number of partitions of a number, provided this number is sufficiently large.
Partitions appear in both mathematics and theoretical physics. When counting so-called coverings of two-dimensional geometric objects (surfaces), mathematicians often encounter specific partition numbers. This type of counting began with the work of mathematician Adolf Hurwitz at the end of the 19th century and has now become a major area of research within both mathematics and physics. Furthermore, in one of his articles, Robbert Dijkgraaf (Mirror Symmetry and Elliptic Curves) described a system from statistical physics in which partitions play an important role. This system is also relevant to Jan Willem's research, as his work generalizes Dijkgraaf's result.
In his research, van Ittersum has studied a specific mathematical function called the q-bracket, which is important for understanding partitions. The idea behind his research is inspired from techniques from statistical physics, where researchers seek the most probable state of a system — for example, a gas in a confined space. However, in van Ittersum's research, the focus is not on a specific state of a system, but on a partition of a number. In broad terms, van Itterstum studied a weighted average of all possible partitions. In his dissertation, he was able to provide a complete picture of when this q-bracket takes a (quasi-)modular form. These quasi-modular forms are mathematical objects with many beautiful properties and symmetries, and can thus provide new insights about partitions.
Modular forms are highly important and complex objects in modern mathematics, but also very elegant. They are of great importance to many fields, both within mathematics and theoretical physics. For example, Andrew Wiles' famous proof of Fermat's Last Theorem is based on modular forms. You should definitely watch this BBC documentary made by Simon Singh about the work of Andres Wiles.
If you want to read a more in depth article about van Ittersum's research you can have a look at the very well written popular summary in his dissertation, or this nice overview (in Dutch) he wrote for the Dutch magazine Nieuw Archief voor Wiskunde. Recently Quantamagazine also published a very nice article about Ramanujan and partitions. Definitely worth reading!
*The feature image is taken from the cover image of the dissertation of Jan Willem van Ittersum.
Two weeks ago I had the pleasure to attend two events where prizes for outstanding research in mathematics were awarded, one of them was the CWI Best Thesis in Applied Math 2024. It was a great pleasure to be there, to chat with (most of) the candidates, and to learn what they have done during their bachelor and master thesis'.
During the last decades, many resources have been invested in quantum computing. This research field has grown immensely and amazing results have been obtained. The plans for the future are highly ambitious, but at the same time, there are serious questions that need to be addressed.
In the city of Utrecht there are four wall paintings of Dutch physisists. Do you know which ones? In this short blog post we want to take you on a virtual bike ride in the city of Utrecht.
The new date for this event is April the 7th 2022 from 19:00 to 21:00 uur. In April the Dutch Academy of Sciences and Arts (KMAW) organizes the "Evening of the Abel prize", a yearly event with as goal to discuss aspects of the research of the latest Abel prize laureates. The event is meant for a […]
